This is a time of year when many of us are running around frantically shopping for gifts. Caught up in the spirit of the holidays, I was looking for some math-themed gift wrap as part of a holiday promotion for Geometiles. In the middle of all the madness, this winter holiday giftwrap caught my eye because of its rotational symmetry. To most people “symmetry” means “mirror symmetry”. But to mathematicians, mirror symmetry is just one of the four types of symmetry used to classify patterns. The other three types of symmetry are rotational, translational, and glide reflection.
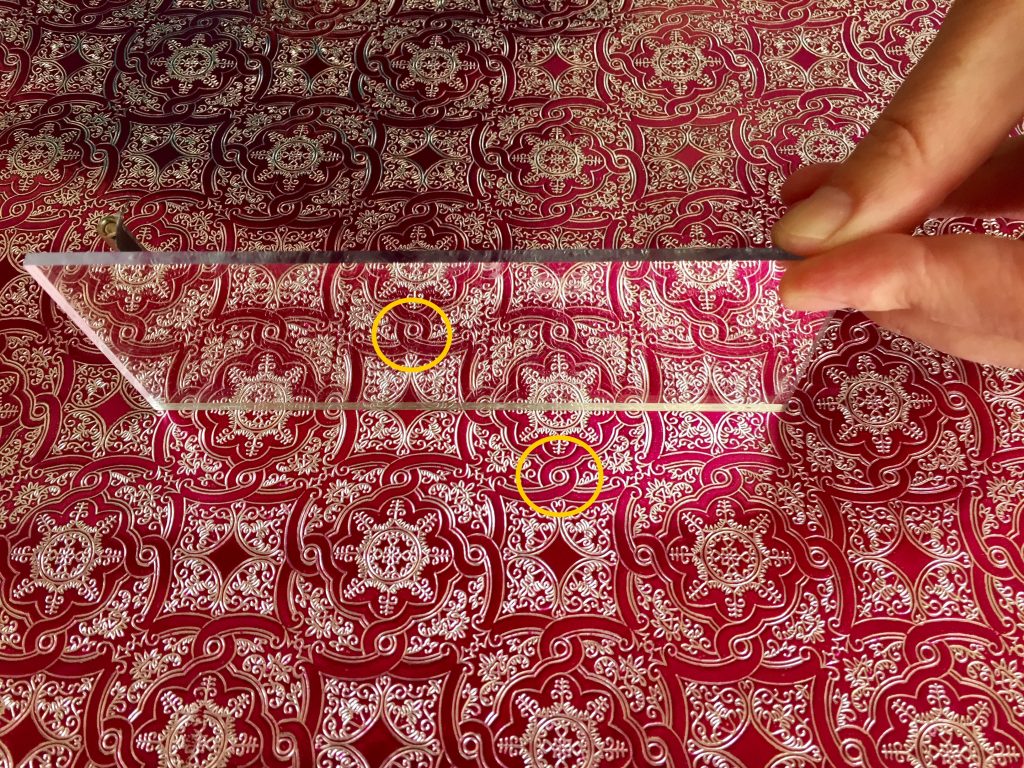
The pattern in the giftwrap in the picture has only rotational symmetry. You can tell it has no mirror symmetry because no matter where you hold an actual mirror, what you see in the reflection is not the same as as the actual pattern of the gift wrap. Take a close look at the small section of the pattern in the yellow circles. Note that they are not the same. If the pattern were mirror-symmetric, they would be identical.
To understand the symmetry of this gift wrap, start by imagining that the giftwrap extends infinitely in all directions. Now think what will happen when you rotate the pattern about the center of the snowflake, the yellow dot, by 90°.

That’s right, it will look exactly the same as if you didn’t rotate it at all. You can keep on going rotating by 90°, and we’ll never know the difference. After 4 rounds of rotating in the same direction, we come back to where we started. Another way to see this is that 360°÷90°=4. We call this rotational symmetry of order 4.
What are other points of rotational symmetry in this giftwrap, and what are their orders? If we rotate about the middle of the square, the pink dot, we find that it’s another point of rotational symmetry of order 4. The last point of rotational symmetry is found at the green dot . We find that if we rotate the whole paper by 180° about this point, it looks the same. Since two 180° rotations bring us to where we started, we say this is a point of rotational symmetry of order 2.

We have exhausted all the points of rotational symmetry that this gift paper has. In the notation developed by John Horton Conway, this gift wrap has a signature of 442, with each number denoting the order of rotational symmetry of the points.

If we combine all the possible symmetries, it turns out, mathematically speaking, that there are only 17 types of gift wrap available in the world!!! To read more about this seemingly shocking fact and lots of related developments, check out the book The Symmetries of Things by Conway, Burgiel, and Goodman-Strauss.
